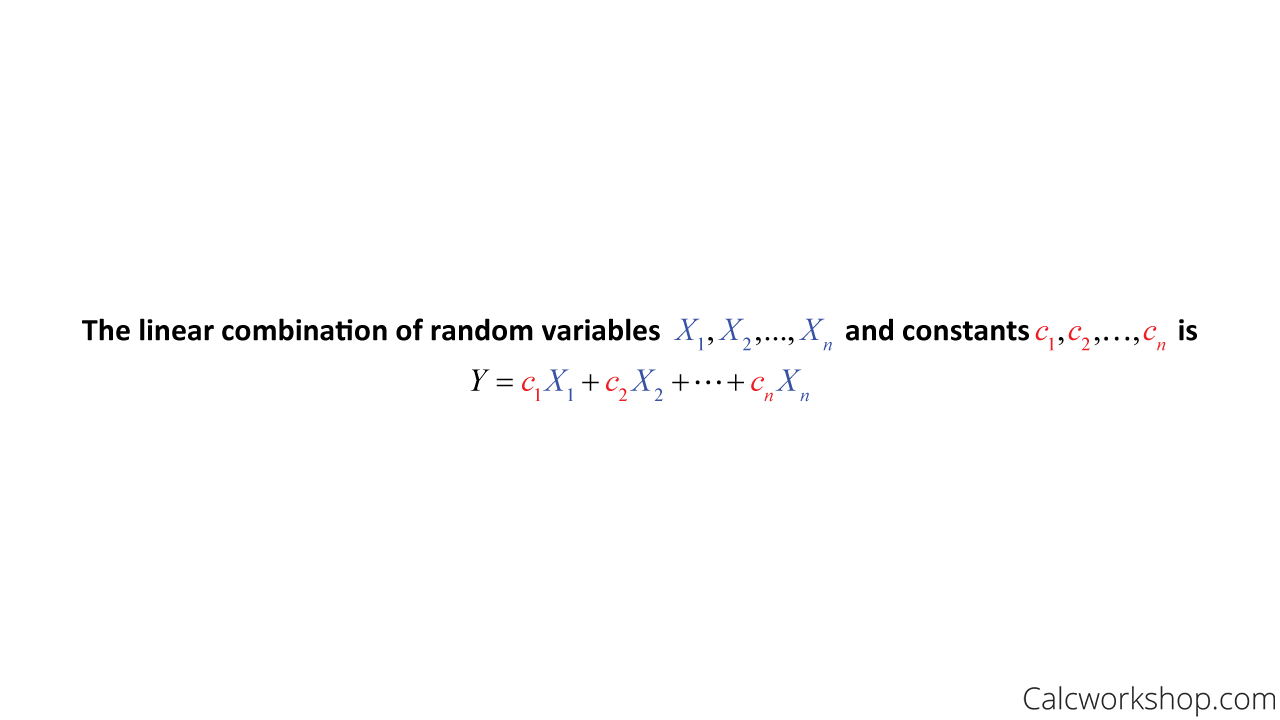Linear Combination Of Poisson Random Variables . Firstly, the sum of multiple poisson. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. If x 1, x 2,., x n are n independent random variables with respective moment. • now, consider the random variable. this distribution has some other interesting properties relevant to linear combinations. Probability & statistics 2 syllabus, written by the. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. Given by f x,y (x,y). • suppose we have two random variables x and y that have a joint p.d.f. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random.
from calcworkshop.com
• now, consider the random variable. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: Given by f x,y (x,y). Probability & statistics 2 syllabus, written by the. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. • suppose we have two random variables x and y that have a joint p.d.f. Firstly, the sum of multiple poisson. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random.
Linear Combination of Random Variables (w/ 9 Examples!)
Linear Combination Of Poisson Random Variables revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: If x 1, x 2,., x n are n independent random variables with respective moment. Given by f x,y (x,y). Probability & statistics 2 syllabus, written by the. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. • suppose we have two random variables x and y that have a joint p.d.f. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: • now, consider the random variable. Firstly, the sum of multiple poisson. this distribution has some other interesting properties relevant to linear combinations.
From www.postnetwork.co
Poisson Distribution in Statistics Academy Linear Combination Of Poisson Random Variables • now, consider the random variable. • suppose we have two random variables x and y that have a joint p.d.f. If x 1, x 2,., x n are n independent random variables with respective moment. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. . Linear Combination Of Poisson Random Variables.
From calcworkshop.com
Linear Combination of Random Variables (w/ 9 Examples!) Linear Combination Of Poisson Random Variables is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. revision notes on 2.2.1 linear combinations of random. Linear Combination Of Poisson Random Variables.
From calcworkshop.com
Linear Combination of Random Variables (w/ 9 Examples!) Linear Combination Of Poisson Random Variables an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: If x 1, x 2,., x n are n independent random variables with respective moment. Given by f x,y (x,y). •. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT Lecture 2 PowerPoint Presentation, free download ID2319982 Linear Combination Of Poisson Random Variables • suppose we have two random variables x and y that have a joint p.d.f. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. If x 1, x. Linear Combination Of Poisson Random Variables.
From www.youtube.com
Statistics Probability 16 Simple Poisson Random Variable Example YouTube Linear Combination Of Poisson Random Variables this distribution has some other interesting properties relevant to linear combinations. If x 1, x 2,., x n are n independent random variables with respective moment. Firstly, the sum of multiple poisson. • suppose we have two random variables x and y that have a joint p.d.f. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT 501 Networking Theory & Fundamentals PowerPoint Linear Combination Of Poisson Random Variables Probability & statistics 2 syllabus, written by the. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: Firstly, the sum of multiple poisson. • now, consider the random variable. this. Linear Combination Of Poisson Random Variables.
From studylib.net
Linear Combination of Two Random Variables Linear Combination Of Poisson Random Variables • now, consider the random variable. this distribution has some other interesting properties relevant to linear combinations. Probability & statistics 2 syllabus, written by the. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in. Linear Combination Of Poisson Random Variables.
From www.postnetwork.co
Poisson Distribution in Statistics Academy Linear Combination Of Poisson Random Variables this distribution has some other interesting properties relevant to linear combinations. Firstly, the sum of multiple poisson. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: Probability & statistics 2 syllabus, written by the. the convolution/sum. Linear Combination Of Poisson Random Variables.
From www.youtube.com
Linear Combination of Multiple Random Variables Example YouTube Linear Combination Of Poisson Random Variables revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. Probability & statistics 2 syllabus, written by the. an easy. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT Poisson random variable PowerPoint Presentation, free download Linear Combination Of Poisson Random Variables the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. Firstly, the sum of multiple poisson. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: suppose. Linear Combination Of Poisson Random Variables.
From andymath.com
Poisson Distribution Linear Combination Of Poisson Random Variables Firstly, the sum of multiple poisson. • now, consider the random variable. If x 1, x 2,., x n are n independent random variables with respective moment. • suppose we have two random variables x and y that have a joint p.d.f. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms. Linear Combination Of Poisson Random Variables.
From calcworkshop.com
Linear Combination of Random Variables (w/ 9 Examples!) Linear Combination Of Poisson Random Variables • suppose we have two random variables x and y that have a joint p.d.f. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. • now, consider the random variable. Probability & statistics 2 syllabus, written by the. revision notes on 2.2.1 linear combinations of random variables for the. Linear Combination Of Poisson Random Variables.
From www.scribd.com
Additive Properties of Two Independent Poisson Random Variables Linear Combination Of Poisson Random Variables this distribution has some other interesting properties relevant to linear combinations. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. • now, consider the random variable. Probability & statistics 2 syllabus, written by the. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT Functions of Random Variables PowerPoint Presentation, free Linear Combination Of Poisson Random Variables this distribution has some other interesting properties relevant to linear combinations. Firstly, the sum of multiple poisson. • now, consider the random variable. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. the convolution/sum of probability distributions arises in probability theory and statistics as the. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT Random Variables PowerPoint Presentation, free download ID6390816 Linear Combination Of Poisson Random Variables Probability & statistics 2 syllabus, written by the. If x 1, x 2,., x n are n independent random variables with respective moment. is there any result about a linear combination of two independent poisson random variables $a_{1} x_1+a_2 x_2$. an easy way to demonstrate this is by using the property of moment generating functions that says for. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT Poisson random variable PowerPoint Presentation, free download Linear Combination Of Poisson Random Variables revision notes on 2.2.1 linear combinations of random variables for the cie a level maths: the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms of. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. Probability & statistics 2 syllabus, written by the. • suppose. Linear Combination Of Poisson Random Variables.
From www.slideserve.com
PPT Functions of Random Variables PowerPoint Presentation, free Linear Combination Of Poisson Random Variables Firstly, the sum of multiple poisson. • now, consider the random variable. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. Probability & statistics 2 syllabus, written by the. an easy way to demonstrate this is by using the property of moment generating functions that says for two independent random. • suppose. Linear Combination Of Poisson Random Variables.
From calcworkshop.com
Linear Combination of Random Variables (w/ 9 Examples!) Linear Combination Of Poisson Random Variables Firstly, the sum of multiple poisson. suppose \(x_1, x_2, \ldots, x_n\) are \(n\) independent random variables with means \(\mu_1,\mu_2,\cdots,\mu_n\) and. • suppose we have two random variables x and y that have a joint p.d.f. • now, consider the random variable. the convolution/sum of probability distributions arises in probability theory and statistics as the operation in terms. Linear Combination Of Poisson Random Variables.